Computational Materials Science Group

Bálint Korbuly¹, Tamás Pusztai¹, Hervé Henry², Mathis Plapp², Markus Apel³, László Gránásy^1,4

¹Institute for Solid State Physics and Optics, Wigner Research Centre for Physics, P.O. Box 49, Budapest H-1525, Hungary
²Laboratoire Physique de la Matière Condensée, École Polytechnique, CNRS, Université Paris-Saclay, 91128 Palaiseau Cedex, France
³Access e.V., Intzestr. 5, 52072 Aachen, Germany
⁴BCAST, Brunel University, Uxbridge, Middlesex, UB8 3PH, United Kingdom

In the literature, contradictory results have been published regarding the form of the limiting (long-time) grain size distribution (LGSD) that characterizes the late stage grain coarsening in two-dimensional and quasi-two-dimensional polycrystalline systems. While experiments and the phase-field crystal (PFC) model (a simple dynamical density functional theory) indicate a lognormal distribution, other works including theoretical studies based on conventional phase-field simulations that rely on coarse grained fields, like the multi-phase-field (MPF) and orientation field (OF) models, yield significantly different distributions. In a recent work, we have shown that the coarse grained phase-field models (whether MPF or OF) yield very similar limiting size distributions that seem to differ from the theoretical predictions. Herein, we revisit this problem, and demonstrate in the case of OF models [by R. Kobayashi et al., Physica D 140, 141 (2000) and H. Henry et al. Phys. Rev. B 86, 054117 (2012)] that an insufficient resolution of the small angle grain boundaries leads to a lognormal distribution close to those seen in the experiments and the molecular scale PFC simulations. Our work indicates, furthermore, that the LGSD is critically sensitive to the details of the evaluation process, and raises the possibility that the differences among the LGSD results from different sources may originate from differences in the detection of small angle grain boundaries.

Frigyes Podmaniczky¹, Gyula Tóth², Tamás Pusztai¹, László Gránásy^1,3

¹Institute for Solid State Physics and Optics, Wigner Research Centre for Physics, P.O. Box 49, Budapest H-1525, Hungary
²Department of Mathematical Sciences, Loughborough University, Loughborough, Leicestershire, LE11 3TU, U.K.
³BCAST, Brunel University, Uxbridge, Middlesex, UB8 3PH, United Kingdom

The first order phase transitions, like freezing of liquids, melting of solids, phase separation in alloys, vapor condensation, etc., start with nucleation, a process in which internal fluctuations of the parent phase lead to formation of small seeds of the new phase. Owing to different size dependence of (negative) volumetric and (positive) interfacial contributions to work of formation of such seeds, there is a critical size, at which the work of formation shows a maximum. Seeds that are smaller than the critical one decay with a high probability, while the larger ones have a good chance to grow further and reach a macroscopic size. Putting it in another way, to form the bulk new phase, the system needs to pass a thermodynamic barrier via thermal fluctuations. When the fluctuations of the parent phase alone lead to transition, the process is called homogeneous nucleation. Such a homogeneous process is, however, scarcely seen and requires very specific conditions in nature or in the laboratory. Usually, the parent phase resides in a container and/or it incorporates floating heterogeneities (solid particles, droplets, etc.). The respective foreign surfaces lead to ordering of the adjacent liquid layers, which in turn may assist the formation of the seeds, a process termed heterogeneous nucleation. 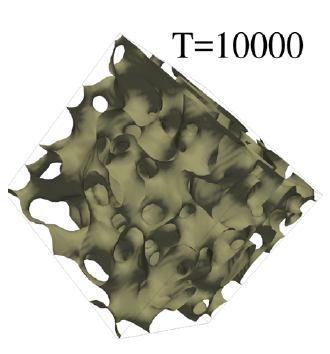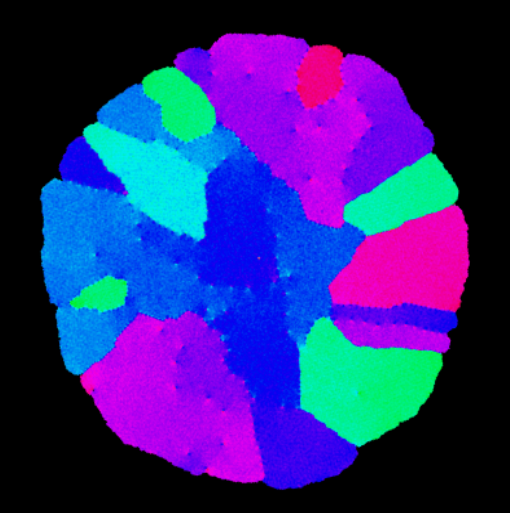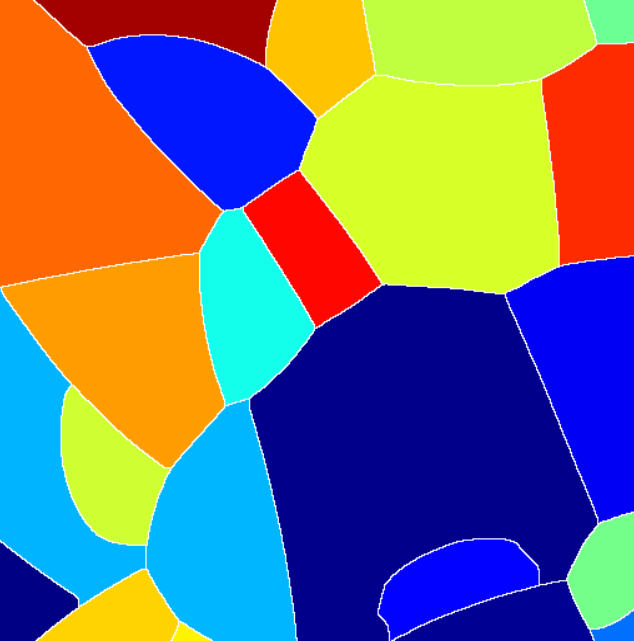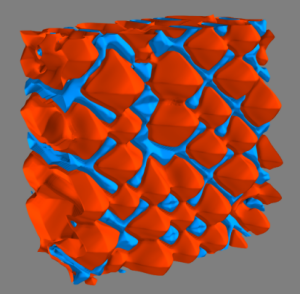Herein, we review how the phase-field techniques contributed to the understanding of various aspects of crystal nucleation in undercooled melts, and its role in microstructure evolution. We recall results achieved using both conventional phase-field techniques that rely on spatially averaged (coarse grained) order parameters in capturing the phase transition, as well as molecular scale phase-field approaches that employ time averaged fields, as happens in the classical density functional theories, including the recently developed phase-field crystal models.

László Gránásy^1,2, Frigyes Podmaniczky¹, Gyula Tóth³

¹Institute for Solid State Physics and Optics, Wigner Research Centre for Physics, P.O. Box 49, Budapest H-1525, Hungary
²BCAST, Brunel University, Uxbridge, Middlesex, UB8 3PH, United Kingdom
³Department of Mathematical Sciences, Loughborough University, Loughborough, Leicestershire, LE11 3TU, U.K.

Structural aspects of crystal nucleation in undercooled liquids are explored using a nonlinear hydrodynamic theory of freezing proposed recently, which is based on combining fluctuating hydrodynamics with the phase-field crystal (PFC) theory. It will be shown that unlike the usual PFC models of diffusive dynamics, within the hydrodynamic approach not only the homogeneous and heterogeneous nucleation processes are accessible, but also growth front nucleation, which leads to the formation of differently oriented grains at the front in highly undercooled systems. Formation of dislocations at the solid-liquid interface and the interference of density waves ahead of the crystallization front are responsible for the appearance of new orientations.

Frigyes Podmaniczky¹, Gyula Tóth², György Tegze¹, Tamás Pusztai¹, László Gránásy^1,3

¹Institute for Solid State Physics and Optics, Wigner Research Centre for Physics, P.O. Box 49, Budapest H-1525, Hungary
²Department of Mathematical Sciences, Loughborough University, Loughborough, Leicestershire, LE11 3TU, U.K.
³BCAST, Brunel University, Uxbridge, Middlesex, UB8 3PH, United Kingdom

We review recent advances made in modeling heteroepitaxy, two-step nucleation, and nucleation at the growth front within the framework of a simple dynamical density functional theory, the Phase-Field Crystal (PFC) model. The crystalline substrate is represented by spatially confined periodic potentials. We investigate the misfit dependence of the critical thickness in the StranskiKrastanov growth mode in isothermal studies. Apparently, the simulation results for stress release via the misfit dislocations fit better to the PeopleBean model than to the one by Matthews and Blakeslee. Next, we investigate structural aspects of two-step crystal nucleation at high undercoolings, where an amorphous precursor forms in the first stage. Finally, we present results for the formation of new grains at the solid-liquid interface at high supersaturations/supercoolings, a phenomenon termed Growth Front Nucleation (GFN). Results obtained with diffusive dynamics (applicable to colloids) and with a hydrodynamic extension of the PFC theory (HPFC, developed for simple liquids) will be compared. The HPFC simulations indicate two possible mechanisms for GFN.