Netta Hendler1,2, Elad Mentovich1,2, Bálint Korbuly3, Tamás Pusztai3, László Gránásy3,4, Shachar Richter1,2
1Department of Materials Science and Engineering, Faculty of Engineering, Tel Aviv University, Ramat Aviv, Tel Aviv 69978, Israel
2Center for Nanoscience and Nanotechnology, Tel Aviv University, Ramat Aviv, Tel Aviv 69978, Israel
3Institute for Solid State Physics and Optics, Wigner Research Centre for Physics, P.O. Box 49, Budapest H-1525, Hungary
4BCAST, Brunel University, Uxbridge, Middlesex, UB8 3PH, United Kingdom
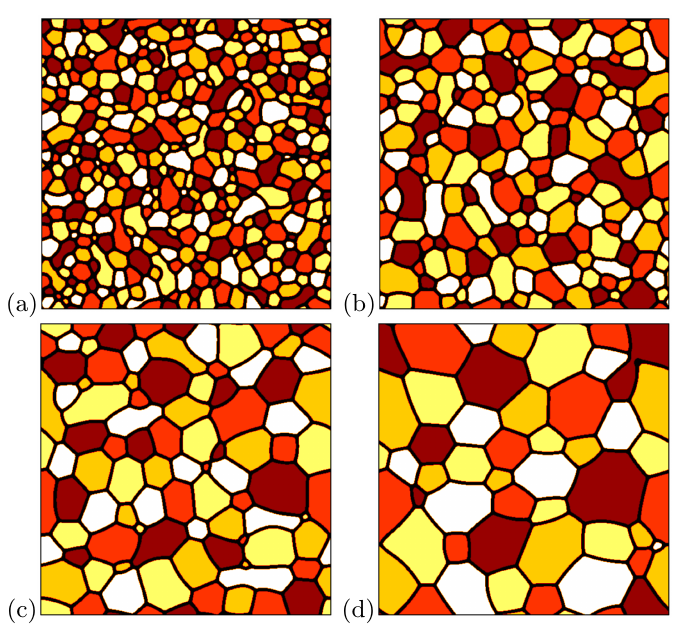
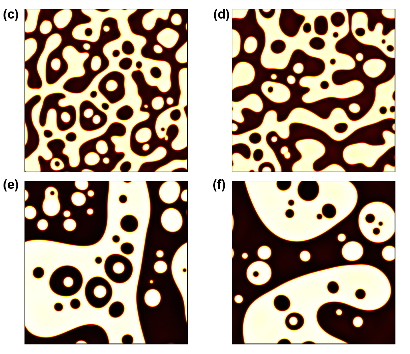
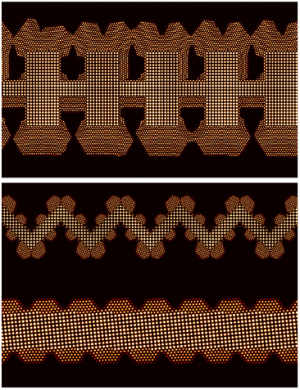
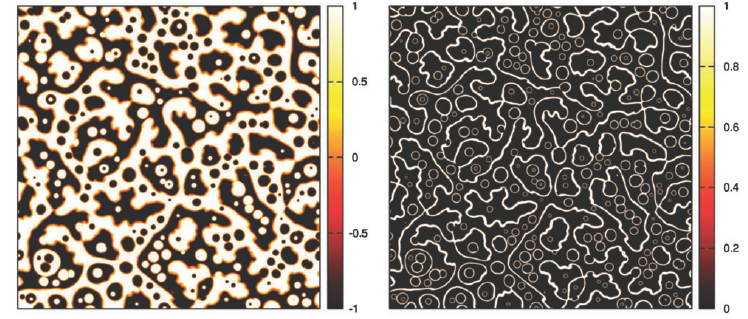
Multi-hierarchical self-assembly (MHSA) is a key process responsible for the spontaneous formation of many complex structures. However, because of the complexity of the process, the underlying mechanism remains largely unclear. Thus, a deeper understanding of MHSA is required, especially for the preparation of MHSA systems via bottom-up methodologies. We show here, experimentally and theoretically, that the complex-formation MHSA of peptide nanotube films can be controlled solely by manipulating the experimental parameter of humidity. Furthermore, we identify growth-front nucleation (GFN; the formation of new grains at the perimeter) as the physical background for the observed morphological transitions by correlating experimental observations with phase-field modeling of the morphological evolution. Our findings indicate a simple way to control multi-hierarchical morphologies, crucial for the employment of bottom-up techniques in constructing complex structures for practical applications.